The Department of Mathematics is staffed with faculty members who are more than just impassioned mentors and educators; they are well respected leaders in their field. Many of the faculty engage in research. Below is a sampling of some of the varied areas in which Monmouth mathematics professors apply their theoretical knowledge in the practical world.
Dr. Laura Turner, Assistant Professor

The History of Mathematics
My research treats the history of mathematics in the late 19th and early 20th centuries. In my work, I focus on not only the origin, evolution, and reception of mathematical ideas, but also on how mathematics is communicated (and to whom, and why), and the different ways in which mathematics is impacted by broader cultural, social, and political issues. I am particularly interested in the history of complex analysis in the advent of set theory; the histories of mathematical journals and congresses; the history of women in mathematics; and the internationalization and nationalization of mathematics around the time of the First World War.
More recently, I have been working on a project involving the origins of our axioms for various mathematical structures, and a related project concerning the ways in which axiom systems are connected to broader educational concerns in the early 1900s. If you’re interested in learning more about the history of mathematics, don’t hesitate to stop by my office!
Dr. Francis Valiquette, Assistant Professor
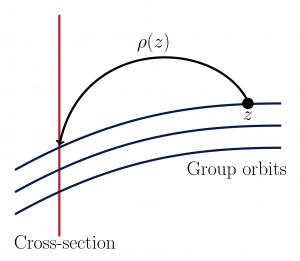
Applications of Moving Frames in Geometry
My research interests lie at the interface of applied mathematics, differential geometry, and discrete geometry. Specifically, I am interested in the theory of transformation groups and the method of (equivariant) moving frames, and their applications to differential equations and their finite difference approximations. In the recent years, have been using the moving frame techniques to solve differential and difference equations that admit a group of Lie point symmetries. I am also interested in symmetry-preserving numerical methods, the study of geometric submanifold flows, and the method of equivalence. If you’d like to learn more about what I do, feel free to stop by my office!
Read more about Professor Valiquette’s research at Francis Valiquette, PhD
Dr. Joseph Coyle, Professor
Numerical Analysis
My research falls into the broad category of numerical analysis. In particular, I have an interest in numerical approximations of wave phenomena or scattering theory. Scattering theory can be divided into two categories: forward and inverse. In the forward problem, the main goal is to compute the scattered wave given an incident field and the medium in which the wave travels. In the inverse problem, one tries to determine the object that actually scattered the wave given the incident and scattered field. Recently I have concentrated my efforts on the forward problem looking at discontinuous Galerikn methods and preconditioning techniques.

Dr. Torrey Gallagher, Assistant Professor
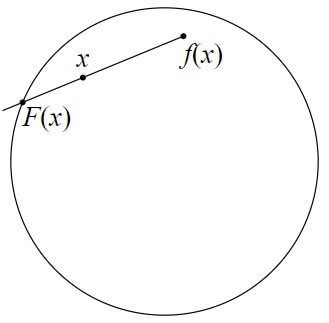
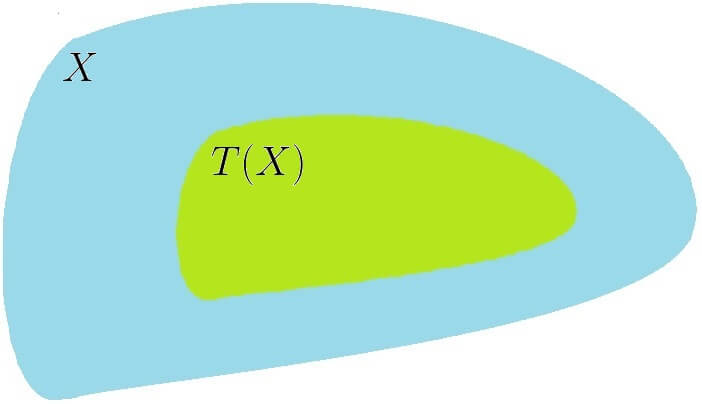
Fixed Point Theory
My research is in an area called fixed point theory. The big idea is to determine which broad classes of functions have fixed points; that is, which functions that have inputs that aren’t changed by applying the function. Somehow, knowing which functions have (or don’t have) fixed points can tell you quite a lot of useful geometric and topological information, and this is what I find most intriguing. For more detailed information, feel free to visit my research page where you can find information about my personal research as well as undergraduate research projects, or stop by my office to chat!

Dr. Richard Bastian, Lecturer
Statistical Analysis of Veterinary or Medical Procedures
Veterinary and medical procedures often balance therapeutic results against risk of side effects; moreover, practitioners often tweak procedures to minimize side effects. Statistical study of the efficacy of these changes helps assess the costs and benefits and facilitates publication of studies. Several projects involving mathematics for statistical analysis of efficacy of veterinary or medical procedures are being conducted. In addition to research involving statistical analysis and interpretation of data, students in my lab learn the basics of client communication and other essential entrepreneurship skills necessary for developing their own consulting practice. Potential projects in consultations with a veterinary practice include “Urinary Incontinence in Spayed Dogs” and “Regenerative Stem Cell Therapy in Dogs”. Consulting projects in conjunction with a urologist include “Serum Prostate Specific Antigen Levels as Predictors of Prostate Cancer”

Dr. Betty Liu, Professor
Partial Differential Equations
My primary research area is numerical solutions of partial differential equations. My research interests are in the fields of numerical analysis, partial differential equations, scientific computation, mathematical modeling, and computational fluid dynamics. I have recently been concentrating on the following two fields of research: numerical analysis and computer simulation. For numerical analysis, I develop numerical methods to approximate the solutions of partial differential equations, prove the unique solvability of the numerical algorithms, and carry out the error analysis of the numerical solutions. For computer simulation, I develop 3D mathematical models to simulate the blood flows in human atherosclerotic arteries, to study the blood flow pattern in curved arteries with or without stenosis, and to investigate the effect of the stenosis on the wall shear stress, the pressure drop, and the flow disturbance. Numerical computations are carried out to allow for simulations of different geometries and flow parameters under the physiological conditions, and the numerical results are analyzed.

Dr. David Marshall, Associate Professor
Algebra and Number Theory
My research is in pure mathematics and has included overlapping areas of algebra, number theory, and geometry. More specifically, I have done work applying Iwasawa theory techniques in algebraic number theory; I have applied arithmetical algebraic geometry techniques to the study of genus 1 curves; and have written pedagogical materials and a textbook for elementary number theory courses. Recently I have been working on projects related to bilinear and quadratic forms over rings and fields of even characteristic, which is an interesting and often overlooked case that is usually omitted from classical treatments on the subject. I’m always interested in hearing about and working on new problems whose solutions are potentially susceptible to the methods of modern algebra, especially those coming from number theory and geometry.

Dr. Wai “Johnny” Pang, Associate Professor
Inverse Problems on Hilbert Spaces
My main research interests are in neighborhood hypothesis and inverse problems on Hilbert spaces. Neighborhood hypothesis is an improved testing method that is both mathematically convenient and practically relevant to replace the usual hypothesis. The advantage is that the asymptotes remain essentially the same with the neighborhood null hypothesis and corresponding alternative reversed. For inverse problems on Hilbert spaces, I focus particularly on improving estimators of inverse function and regression models in Hilbert Spaces. For the past two years, I have also been including student researchers in the above topics with applications.
